19edo vs. Carlos Beta
18 January 2011 Filed in: Tuning
Theory

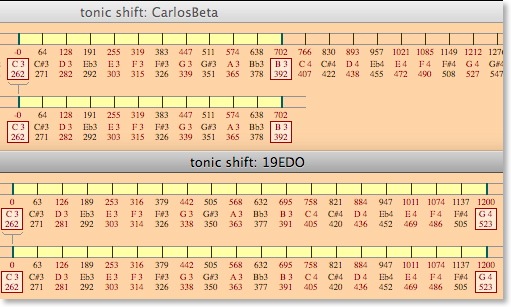
My recent decision of adopting Carlos Beta as a tuning system to investigate prompted some considerations. The first one is its similarities with 19EDO.
There is a vast literature about 19EDO so I went back reading “Multiple Division of the Octave and the Tonal Resources of 19-Tone Temperament” by M. Joel Mandelbaum.
I found something relevant to the relationship between these two tuning systems on pages 11 and 12:
Joseph Yasser at one time suggested an incursion on the hegemony of the octave by treating the perfect fourth or the perfect fifth as a complete identity capable of enclosing a musical system. Such a fourth and fifth he proposed to call a "quartave" and a "quintave" respectively.
This is exactly what happens, for example, with Carlos Alpha, Beta and Gamma where a perfect fifth is considered a “complete identity capable of enclosing a musical system”.
I even created a notation system for Carlos Gamma where each perfect fifth interval is identified by the same letter name. Unaware of the above-mentioned Yasser’s article I had thought of calling this framework interval “quintave” but had discarted it in favor of “diapente”.
A few more paragraphs by Mandelbaum (on the same pages):
In addition to proposals to eliminate the octave or to replace it as the primary "identity" in music, there have been a few suggestions of recent origin that the octave should not be tuned precisely.
A number of studies have shown that at the highest and lowest ranges of hearing the interval 2:1 must be stretched a substantial amount to produce the psychological effect associated with the octave. The suggestion has recently been made that 2:1 be slightly enlarged throughout the pitch continuum to produce its most agreeable effect. It has also been proposed that the octave be made smaller.
Bingo!
It remains to be seen if Mandelbaum would consider a stretched octave of 1,212.467 cents “slightly enlarged” (as with Carlos Beta).
So, I started thinking about Carlos Beta from a “stretched octave” point of view. It can be represented as a chain of 19 perfect fifth intervals:
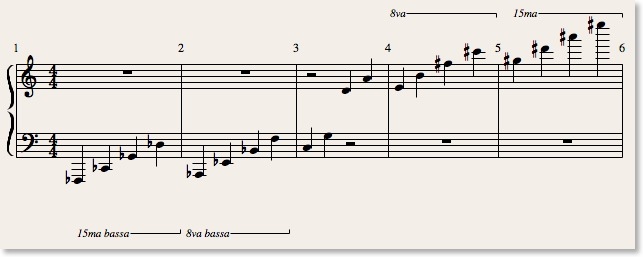
Fb-Cb-Gb-Db-Ab-Eb-Bb-F-C-G-D-A-E-B-F#-C#-G#-D#-A#-(E#)
exceeding 11 octaves by 137.145 cents:
19 x 701.955 = 13,337.145 (11 x 1,200 = 13,200 + 137.145)
Dividing 13,337.145 cents by 11 we get 1,212.467 cents (Carlos’ Beta “octave” )
Dividing 1,212.467 cents by 19 we get 63.814 cents (1 Carlos Beta Unit).
This analysis is based on a similar one X.J.Scott did for his “superpythagorean” scale (a chain of 12 perfect fifths divided by 7 octaves resulting in a “slightly enlarged” octave 1203.351 cents wide).
Here there is a table comparing how closely the two tuning systems match harmonic ratios:
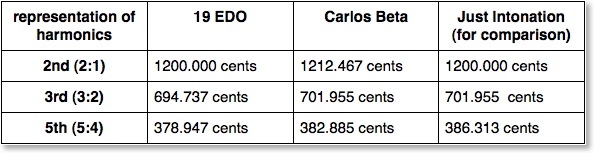
I will end quoting Wendy Carlos from her above-mentioned article:
Beta sounds even better than 19-step Equal, which is troubled by a fairly flat major third of less than 379 cents, which sounds rather anemic to our ears, brought up as we are in a very sharp major third world of E.T. Melodically it's quite impossible to hear much difference between Beta and 19-tone Equal.
X.J.S commented:
Cool article.
Wow, never saw that Yasser quote before, and from 1945, great find.

My recent decision of adopting Carlos Beta as a tuning system to investigate prompted some considerations. The first one is its similarities with 19EDO.
There is a vast literature about 19EDO so I went back reading “Multiple Division of the Octave and the Tonal Resources of 19-Tone Temperament” by M. Joel Mandelbaum.
I found something relevant to the relationship between these two tuning systems on pages 11 and 12:
Joseph Yasser at one time suggested an incursion on the hegemony of the octave by treating the perfect fourth or the perfect fifth as a complete identity capable of enclosing a musical system. Such a fourth and fifth he proposed to call a "quartave" and a "quintave" respectively.
This is exactly what happens, for example, with Carlos Alpha, Beta and Gamma where a perfect fifth is considered a “complete identity capable of enclosing a musical system”.
I even created a notation system for Carlos Gamma where each perfect fifth interval is identified by the same letter name. Unaware of the above-mentioned Yasser’s article I had thought of calling this framework interval “quintave” but had discarted it in favor of “diapente”.
A few more paragraphs by Mandelbaum (on the same pages):
In addition to proposals to eliminate the octave or to replace it as the primary "identity" in music, there have been a few suggestions of recent origin that the octave should not be tuned precisely.
A number of studies have shown that at the highest and lowest ranges of hearing the interval 2:1 must be stretched a substantial amount to produce the psychological effect associated with the octave. The suggestion has recently been made that 2:1 be slightly enlarged throughout the pitch continuum to produce its most agreeable effect. It has also been proposed that the octave be made smaller.
Bingo!
It remains to be seen if Mandelbaum would consider a stretched octave of 1,212.467 cents “slightly enlarged” (as with Carlos Beta).
So, I started thinking about Carlos Beta from a “stretched octave” point of view. It can be represented as a chain of 19 perfect fifth intervals:


Fb-Cb-Gb-Db-Ab-Eb-Bb-F-C-G-D-A-E-B-F#-C#-G#-D#-A#-(E#)
exceeding 11 octaves by 137.145 cents:
19 x 701.955 = 13,337.145 (11 x 1,200 = 13,200 + 137.145)
Dividing 13,337.145 cents by 11 we get 1,212.467 cents (Carlos’ Beta “octave” )
Dividing 1,212.467 cents by 19 we get 63.814 cents (1 Carlos Beta Unit).
This analysis is based on a similar one X.J.Scott did for his “superpythagorean” scale (a chain of 12 perfect fifths divided by 7 octaves resulting in a “slightly enlarged” octave 1203.351 cents wide).
Here there is a table comparing how closely the two tuning systems match harmonic ratios:

I will end quoting Wendy Carlos from her above-mentioned article:
Beta sounds even better than 19-step Equal, which is troubled by a fairly flat major third of less than 379 cents, which sounds rather anemic to our ears, brought up as we are in a very sharp major third world of E.T. Melodically it's quite impossible to hear much difference between Beta and 19-tone Equal.
X.J.S commented:
Cool article.
Wow, never saw that Yasser quote before, and from 1945, great find.


